Holding up a Mirror to Mathematics
Katie Steckles
This month, a new exhibition will be visiting the MAINS in Heidelberg – on the mathematical secrets behind mirrors. The exhibition, running from February 12 to August 27, 2023, contains a variety of interactive exhibits allowing you to explore the fascinating world of mirrors – from kaleidoscopes to magic tricks, and plenty of mathematical ideas including symmetry and infinity.
Mirrors are familiar household objects – they can be found in many rooms of the average house – and you’re likely used to seeing your own face in one. But sometimes the nature of mirrors can trick us. While you’ll have to visit the exhibit to see this for yourself, here are some mathematical puzzles and thought experiments you can try in the meantime. Take a minute to think about each of these, and read on once you’re satisfied.
- Imagine a mirror mounted vertically on the wall in front of you – one in which you can see yourself, from the top of your head down to a point on your waist. Now think what would happen if you step backwards, away from the mirror. Can you see more of yourself, or less of yourself?
- Images seen in a mirror are reflected – if you hold up a piece of paper with writing on, the text in the mirror will be backwards. But it will still be the right way up! So why do mirrors reflect left-to-right, but not top-to-bottom?
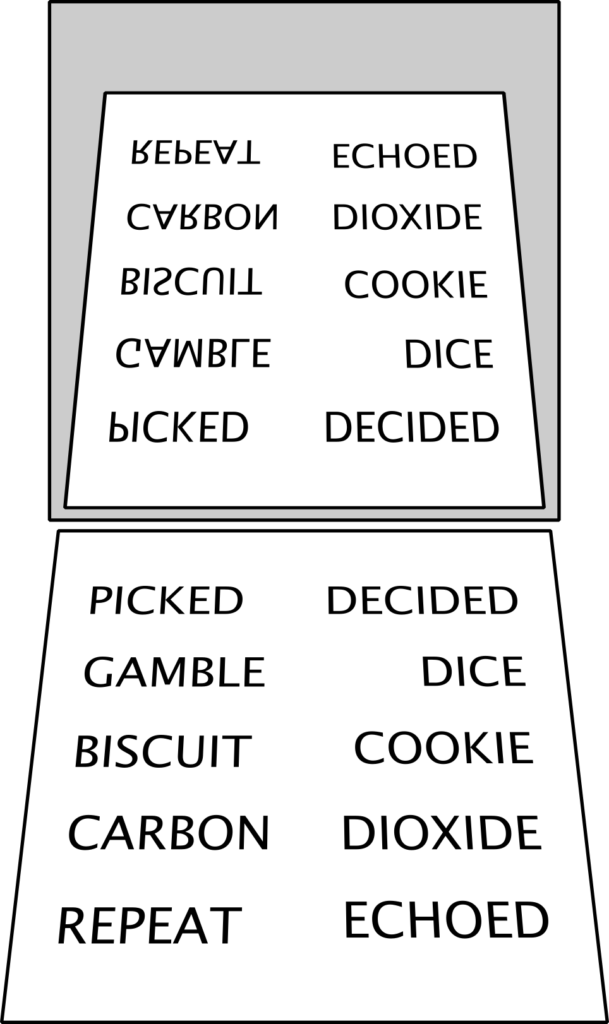
- Here’s a selection of English words, in connected pairs.
If I place a mirror along the bottom edge of this sheet, and look at the words in the mirror, here’s what I see. Try to read the words:
What would happen if I placed a mirror along the top edge of the card instead? If you’ve got a small mirror handy, you can print a copy of my word list (or bring it up on a screen) and hold the mirror against the bottom edge, then the top edge. Can you explain what you see? (If you don’t have access to a mirror, here’s a photo of what happens when the mirror is at the other end – but it’s nicer to see it for yourself).
Smoke and Mirrors
The mathematics behind mirrors is partly about physics – the way light bounces off a reflective surface will determine what you see when you look in a mirror. But it also relies on symmetry: mathematical operations you can perform on a shape or object which reflect it, through a central line which stays in place, swapping the two sides across the line.
- Mirror Mirror, on the Wall
Our first problem here relies on purely angles and physics. The ray of light bouncing off you, hitting the mirror and being reflected back into your eye will determine what you can see, and light rays behave in a mathematically predictable way: the angle it bounces off the mirror is the same angle it hit the mirror at, so the lowest point on your waist you can see in the mirror will be as far below the bottom of the mirror as your eyes are above the bottom of the mirror.
Thinking about this question, you might suspect that the amount of you visible in the mirror would increase as you walk backwards, since you’re further away, and therefore smaller relative to the mirror. You might also reasonably think you’d be able to see less, since your eyes are further away and the mirror is smaller relative to your eyes, so the angle you can see gets tighter, and you won’t be able to see as far down.
It turns out both of these are right! The amount of yourself you can see, if you step backwards away from the mirror, will actually not change at all. You’ll still be able to see the same point on your waist you could see before, and no more or no less. If you’re not convinced, Ben Sparks has made a great Geogebra demonstration which allows you to change the distance away from the mirror, and the height and size of the mirror, so you can play with it until you’re convinced.
- It’s Just a Jump to the Left, and Then a Step to the Right
The mystery of why mirrors reflect left-right but not up-down has plagued anyone who is aware of how light behaves around reflective surfaces and subsequently had this pointed out to them. Light bouncing off the mirror doesn’t know which way up the mirror is, so how could it know to change the direction of the text on a piece of paper horizontally but not vertically?
It’s not something you would necessarily notice, since you’re used to looking in mirrors at mostly symmetrical objects like your own face – and you quickly get used to the fact that putting up your right hand will cause your reflection to put the hand up that you can see on your right (even though, if you were the person in the mirror, it would be your left hand).
The crucial thing here is that people forget that they have made some decisions too in the mirroring process. When you hold up a piece of paper with writing on it in front of you, you don’t reflect it: you’ll be moving a physical object around in space, and performing a rotation. And crucially, you choose which way to rotate it. If you were showing some text to a real person opposite you, it would be nonsense for you to flip the card you’re looking at over vertically and show it to them upside down.
Similarly, when you look in a mirror you turn the paper around left-to-right, rather than top-to-bottom. If you did flip it over top-to-bottom, the writing would be reflected vertically, not horizontally. Similarly, when you imagine the person in the mirror holding up their left hand instead of their right, the process you need to go through to map yourself onto them – if the mirror was instead a glass window set into a narrow wall – would be to walk around to the other side of the wall and rotate yourself (horizontally) so you’re facing the other way.
This is why the left and right hands are assuming opposite roles, and why mirrors appear to reflect left-to-right – it’s not the mirror that’s chosen that direction, it’s you!
- I’m Looking at the Words in the Mirror
Placing a mirror along the bottom edge of the card and looking at its reflection means you’re seeing the card the right way up – the part nearest to you on the table is furthest away in the mirror – but it’s reflected (as discussed above) left-to-right. Unless you’re skilled at reading mirror writing, you might struggle to make out all of the words as fluently as you would if you were reading them directly from the paper, the right way round.
But in the second case (shown to the right), you might find that the words – or, some of them at least – become much easier to read. It might take you a while to work out the difference between the two sets of words; it’s inconceivable that some words are immune to mirroring! How is it possible they are still the right way round? It turns out: they aren’t – but what you’re seeing here isn’t the kind of reflection you’re expecting.
Reflection symmetries are part of mathematical symmetry groups – I’ve written about groups here previously, and symmetry groups are often used as a simple example. The ‘group’ is the collection of possible symmetries of a shape, and we can think about combining symmetries – ‘adding’ them together within the structure of the group – by simply performing one, then another.
For example, reflecting a square horizontally then reflecting it vertically has the same result as rotating it through 180 degrees. (Try it now, if you’ve got a flat square-ish object in front of you).
Combining a rotation and a reflection will result in a reflection – and when we look at this card upside-down, but in the mirror, that’s exactly what we’re doing. A 180-degree rotation combined with a horizontal reflection gives a vertical reflection, and the view of the card you get is what would happen if you flipped it vertically. You’re so used to seeing writing in mirrors flipped left-to-right, but not top-to-bottom – but if you look carefully, both sets of words (even the unreadable ones) have the letters in the correct order from left-to-right, but they’re upside down.
The difference between the two sets (which you may already have worked out) is that the ones which can now be easily read are made up entirely of letters with vertical symmetry. H, I, O, D, C and K (assuming you choose your typeface carefully) all have symmetry under a top-to-bottom flip and look the same. This means when you filp the word vertically, it just looks like the same word.
It’s worth noting that some letters do have left-to-right symmetry too, including H, T, U and others – but if you flip a whole word horizontally, the order of the letters is also reversed, so it’s still harder to read. This curious mirror trick can be a real hook – especially for younger mathematicians – to get you thinking about exactly how the mirror is transforming what you see.
Mirrors are functional, useful objects we encounter every day, but hopefully this has shown you that there are plenty of interesting mathematical questions and curiosities hiding out of sight. So next time you look in a mirror – whether it’s in your bathroom at home, or in the Heidelberg MAINS exhibition – bear in mind it’s not always as simple as it looks!
The post Holding up a Mirror to Mathematics originally appeared on the HLFF SciLogs blog.